Getting Thor Back from the Moon – Mjolnir Power!

by Matt Brady and Michael Surratt

Well, when you suddenly find that you’re Thor and you’re on the moon, what would you do? You’d want to go back home too.

There’s been a new Thor in the Marvel (comics) Universe for a little while now. Dr. Jane Foster is the new, female version of the God of Thunder. A year or so back, in the (then) new Thor #1, the male Thor found himself unworthy to lift the hammer, Mjolnir and dropped it on the moon. A crowd (of Asgardians) gathered and watched Thor (male) and his father (Odin) try and lift it, but in the end…when everyone had walked away, a mysterious figure – now revealed to be Jane Foster – lifted it, and, like the label says, possessed the power of Thor.

But – in issue #2, that left Foster with a problem. She was on the moon, with no idea how to get back to earth until: “Wait. I’ve seen Thor do this before. You…whip it around really fast like this, right? Then you throw it as hard as you can and you just…hang on!”

And with that, Foster was headed back to earth.

But all of that got us thinking. Assuming that you’re invulnerable, and impervious to the hazards of no atmosphere and space, how do you do that? That is, what’s involved in traveling back from the moon under Mjolnir power and Mjolnir power alone?

It’s all physics.

First off – some assumptions and estimates:

• Mjolnir’s mass. The best explanation we found (okay, the one we like a lot and can work with) is that Thor’s mystical hammer weights 42.3 pounds, which means it has a mass of about 19 kilograms. Yes, we know that Neil deGrasse Tyson said that if it was made out of neutron star matter, it would weigh as much as 300 billion elephants, but we like Matt Shipman’s version better – and it dates back to some ancillary Marvel merchandise – a trading card. Why can’t anyone pick it up? Since it’s not heavy per se, it has to do with whoever’s worthy. No – we’re not invoking magic. We’re saying that it looks like magic, but according to Arthur C. Clarke’s Third Law, that just means it’s crazy advanced science.
• We’re taking the weight of Jane Foster Thor (plus armor clothing and gear) to be about 150 pounds.  We’re working off of Jane-Thor (not cancer patient Jane) being about 135 pounds, with the rest adding about 15 pounds. 150 pounds is about 68 kg. Your mileage may vary, and if it does, put your own numbers in and do the math. It’s fun!
• We’re giving some comic book, “magical” passes on some aspects of Thor’s flight – not worried about how Thor can stand on the moon while twirling her hammer, we’re not worried about re-entry into earth’s atmosphere or her landing. She is Thor after all. She’s tough.
• The only source of kinetic energy (the energy needed to move Thor) would come from Mjolnir. There are no rockets, no strings pulling her, nothing other than the power of a mystic hammer. And given that this is Jane’s first day as Thor, she’s going to be efficient in her use of energy. More on that later.

With our assumptions and estimates in hand, it’s time to look at the larger problem. If you’re on the moon, you’ve got two things to worry about if you want to get home: 1 – where do you have to fly to, relative to the moon? And 2) How much energy do you need to get there?

Let’s start with the first one.

You Don’t Have to Go the Whole Way…

Here’s the thing that we’ve known about coming back from the moon since…well, before we were coming back from the moon. You don’t have to use enough energy to make the entire trip. The most efficient way to get home from the moon is to get to the point along the line between the Earth and Moon’s respective centers of mass where the two gravitational attractions are equal. Just push a shade past that point – towards earth – and earth’s gravity will do the rest for you. You’ll fall back home, without any propulsion. Mother earth will pull you in for a hug.

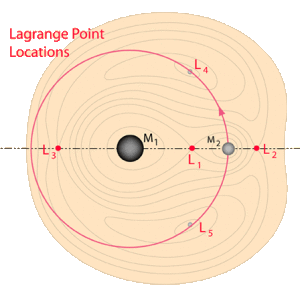
That point has a name – it’s one of the five Lagrange Points between the earth and moon. Specifically, we’re looking at L1.

 

Determining the location of L1 starts with Newton’s Law of Universal Gravitation between homogeneous spherical bodies…

…and builds from there. By the way:

F_{grav =} the force of gravity

G = Gravitational constant, 6.674×10⁻¹¹ N⋅m²/kg²

M, m = masses of the two objects, M is the larger mass, m is the smaller mass.

d = distance between the bodies’ respective centers of mass

Hang on – things are going to get a little mathy. If it gets to be too much, just scroll down to the next picture of Mjolnir and rejoin us there.

If we simultaneously apply Newton’s Second Law in the constant mass case, we can see that the gravitational force is the mass of the small object (m) times the acceleration due to gravity at that location.

Where is the acceleration on any mass between the Earth and Moon equal from both large bodies? We need to know this because if the small mass is just slightly closer to Earth than that point, it will continue to fall toward Earth without any propulsion.

To find this balance point, set the accelerations caused by each of the Earth and Moon equal to each other. That balance point is the First Lagrange Point (L1) in the Earth-Moon system. Divide out the mass of the small body (m) from the expression for Universal Gravitation, and you get:

For any solar system body, the product GM is called the gravitational parameter. The inside front cover of Space Mission Engineering: The New SMAD, Wertz, Everett, and Puschell, Microcosm Press, 2011 provides the two parameter values that we need.

GM_Earth = 3.9860 x 10¹⁴ m³/s² and GM_Moon = 4.9028 x 10¹² m³/s²

Before we start slinging big numbers around, let’s rework the gravitational acceleration equality to get unknowns on the right side of the equal sign and knowns on the left side. Divide both sides by GM_Moon and multiply both sides by d²_Earth-L1 

Now, substitute both known gravitational parameter values and divide them. This results in a non-dimensional ratio of the square of the two distances.

Apply the square root to both sides.

This tells us that the distance from the center of the Earth to L1 is about nine times as far as the distance from the center of the Moon to L1.

Recall from the first page, the two known facts about the distance between the Earth and Moon as well as the distances from each large body to the L1 point? The two distances to L1 sum to the total distance between the Earth and Moon.

Be careful at this point. That computed distance is from the center of mass of the Moon to the L1 point, not the distance from the lunar surface to the L1 point. The Moon has a mean equatorial radius of 1737.5 km, so the distance Thor has to fly is 30,039.5 km away from where she stands on the surface, which is 1737.5 km away from the center of the moon.

So finally, we know where Thor starts and where Thor has to arrive – the L1 point – to then fall the rest of the way to Earth.

So how does she get there?

Let’s talk energy.

Let’s Light this…Hammer

The concept of getting Thor to L1 is simple – she just has to change her energy state, from being on the moon to being at L1. This means we’re talking about Thor’s gravitational potential energy. You may remember from a physical science class that:

E_potential = mass x gravity x height

With gravity being equal to the gravitational field of whatever planet you’re on, usually earth at 9.8 m/s². But when you’re flying through space, that formula won’t hold up. As you move farther from the center of mass of a planet, the effect of gravity from that planet (or moon) decreases with distance. It’s time for orbital mechanics.

Astrodynamicists and others in the field of spacecraft mission design use an unusual scheme to analyze these types of problems. They make the zero potential energy state out at an infinite distance from the central mass. The reasons for this are beyond the scope of worrying about Thor and her hammer, but for now, we have to be aware that it means all gravitational potential energy values closer than infinity are negative. Without proof, the relationship for specific gravitational potential energy (energy per unit mass) “e” we will use is the following (where d is the distance from the central body’s center of mass to the smaller object).

M = mass of the moon, 7.35 x 10²² kg
G = Gravitational constant, 6.674×10⁻¹¹ N⋅m²/kg²
Running the numbers then, at the Moon’s surface (converted to meters from kilometers), the specific potential energy is –(4.9028 x 10¹² m³/s² / 1737.5 x 10³ m) = -2.822 x 10⁶ J/kg.

At the position of Earth-Moon L1, the specific potential energy is –(4.9028 x 10¹² m³/s² / 39774 x 10³ m) = -1.233 x 10⁵ J/kg.

Final energy minus initial energy gives us the needed difference, which is 2.699 x 10⁶ J/kg. That energy will come from swinging that hammer on the lunar surface to launch Thor up to Earth-Moon L1.

We’re using 68kg for the mass of Thor, as we mentioned earlier, so multiplying 68kg by the specific potential energy change we arrive at the total kinetic energy that must be applied to the Thor-Hammer system to get to Earth-Moon L1. That product is 183,532,000 Joules or 183.5 Megajoules.

Where does that energy come from? She’s swinging it.

At the beginning of this, we agreed that the only source of kinetic energy would be spinning the hammer and then suddenly redirecting all of that rotational kinetic energy into translational kinetic energy directly away from the lunar surface with Thor hanging on for the ride to Earth-Moon L1. Let’s make a simplifying assumption that the entire hammer mass can be approximated as a point mass at the end of the handle. Let’s also take the rotational length of Thor’s arm and hammer (R) as approximately 1 meter.

This means we need to spin up a 19kg hammer mass (m) in a 1 meter radius circle (R) with sufficient but unknown rotational speed (w) to give us a total of 183.5 MJ of rotational kinetic energy in the hammer. The expression relating all of these parameters to rotational kinetic energy is this:

The only unknown is rotational speed. Rearrange the energy relationship before you substitute in values to obtain this equation for unknown rotational speed.

Unit analysis shows that we will get a pure number per second as the final answer. That means we are getting an answer in radians per second. Substituting in the values 183.5 x 10⁶ J, 1m, and 19kg in their proper places we get a rotational speed of 4395 radians per second.

Let’s jump back to high school math & physics. How many radians are there in one revolution? Two pi. So divide that rotational speed by 2π to get the number of revolutions per second needed to build up the energy needed. The result is 700 revolutions per second (not rpm, but rps!) Good thing there is no sensible atmosphere on the Moon to slow down the hammer by aerodynamic drag (*).

So there you go – to get home from the earth, the most efficient way possible – fly to L1 and let earth’s gravity bring you in, Thor would have to get 30,039.5 km away from the surface. Getting there will cost her 183.5 Megajoules of energy. And how she’s getting all that energy? Swinging Mjolnir at 700 revolutions per second.

Not bad for day one as Thor.

Current issues of Thor can be found at your local comic shop, or purchased as digital editions via Comixology.

(*) – But what if there was? In other words, what if Thor was swinging Mjolnir at 700 rps on earth, with its thick atmosphere of air? The air would push back, and she’d need more force to swing the hammer at a constant 700 rps, that much is easy to figure out. But there’s one other thing that always seems to get left to the wayside when Thor – any Thor – swings their hammer at a high revolutions.

Mjolnir sings.

Swing Mjolnir at 700 rps, so it makes 700 oscillations per second means that, where there’s an atmosphere, the hammer is producing a 700 Hz tone, an F note. “Fa” on the do-re-mi scale. We’d like to think the hammer wobbles a touch and produces a chord, but that might be just us.

For an idea of the pitch – check out the video below: