It’s Raining Kryptonians – Batman’s Revenge

Just remember – if you’re a Kandorian “god,” always have a plan B.

That is, especially if your method of attack – like being high off the ground – could be a liability if exploited correctly by someone with a lot of money, synthetic Kryptonite, a swarm of drones in orbit and the ability to seed clouds and lace the rain with Green Kryptonite.

Yeah – Batman has all of that.

In Dark Knight III #5 (by Frank Miller, Brian Azzarello, Andy Kubert and Klaus Janson) – which is currently in comic shops, or at Comixology here, a group of Kandorians are flying to Gotham City to put down Batman who, as he will, is standing in defiance against the citizens of Kandor. The Kandorians are Kryptonians like Superman, who had been miniaturized for decades until recently (issue #2) when the Atom was tricked into re-growing them. Posing as helpless citizens of Kandor while talking to the Atom, the Kandorians were, in fact, religious zealots who saw earth as theirs for the taking, led by Quar, who sees himself as their god. They wanted earth to fall in line under them.

But Batman said no.

Well, he actually said:

Hence the flock of Kandorians flying over his city, and his need to use synthetic Green Kryptonite to bring them down to earth – literally.

So – when the Kandorians start raining down on Gotham City…what’s that like? How much damage would a falling Kandorian do to the stuff on the streets of Gotham City?

Luckily, we know some physics and stuff, so we can get into The Science of Falling Kryptonians.

(By the way – teachers – this is an example of something I would set up for my students as a homework assignment. They would have to figure out all the particulars of the height of the Kandorians, the energy, the conversions, and all the rest.)

Okay – we need to know a few things before we start really sciencing.

First of all – how high up are the Kandorians? We’ve got two clues, but admittedly we’re going to be making some assumptions.

Clue #1: Barry Allen (The Flash) has been manipulating the weather via supercomputer, adjusting millions of variables in nanoseconds as his brain processes the information faster than any human. He’s trying to make a serious rainstorm by seeding the clouds, so let’s go with the idea that he’s making a perfect storm cloud. There’s thunder too. Let’s go with the rain that falls is coming out of a cumulonimbus cloud that Barry was able to coax out of the atmosphere.

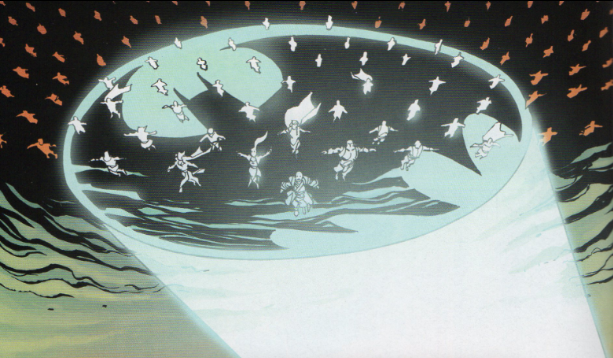
Cumulonimbus clouds are the towering storm clouds that can reach miles into the atmosphere, and often have flat “bottoms” when observed from the ground, which matches the image of the Batsignal being projected on the relatively flat surface of the cloud. An average height for the bottom of a cumulonimbus cloud? Let’s go with 1,000 meters, which is in the accepted range, and agrees with clue #2.

Clue #2: We see the Kandorians in the sky, many roughly at the level of the cloud bottom, illuminated by the Batsignal. Assuming that the point of view of the image of the flying Kandorians is on the ground, the lengths of the Kandorians range from about 1 cm to 2 mm. We can use a formula:

Apparent height = actual height/distance

Where:

Apparent height: how big the thing looks in the sky to you, the observer

Actual height: the true height of the object you’re observing

Distance: The distance between you and the object

As the formula stands now, it’s easy to see that as the distance between you and the object (the denominator) gets bigger, the apparent height gets smaller. Things look smaller as you move farther away from them.

We know the apparent height and we can approximate the average actual height (let’s use 2 meters – Kandorians are a healthy stock), and let’s use the 2 mm as our apparent height. We want to get an idea of distance. So we need to rearrange the formula to solve for distance (and change our millimeters to meters):

Distance = actual height/apparent height

Distance = 2 meters/.002 meters = 1000 meters.

Good enough – we’ll take the height at which the Kandorians are flying at 1000 meters.

But we’re not here to talk about flying Kandorians. We’re here to talk about free falling Kandorians, and how much damage they do when they land. And we want to figure it out in the simplest way possible.

First off – we’re not going to ignore air resistance completely. The Kandorians are falling through air. Air offers resistance to anything falling through it (put your hand outside the car window as you’re traveling), and that resistance is due to a few things: 1) the surface area the falling object presents to the air, 2) the air’s density, 3) the drag coefficient, which depends upon the shape of the falling object. As a result of all three, the air pushes back on the thing that’s falling, and, if the thing is falling long enough, the force of the air pushing back on the falling thing will equal the weight of the thing that’s falling.

Up to this point, the falling thing has been accelerating – falling faster and faster per second. When something is falling near earth’s surface (like the Kandorians) the acceleration is 9.8 meters/second/second – each second, the falling object adds another 9.8 m/s to its speed. But when the push of the air upward equals the weight of the falling object, there’s no more acceleration. The velocity stops growing, or terminates – the falling object as reached its terminal velocity. It will fall downward at this speed for the remainder of its fall.

If you’re a regular The Science Of… reader and this sounds familiar, it should – we spoke about it with the Invisible Woman a few months back.

How does this apply to our falling Kandorians? We have to figure out if they reach terminal velocity during their fall from 1000 meters. To do that, we need to again, make some assumptions to simplify things and crunch some numbers.

Normal terminal velocity checks in at about 120 miles/hour (53.6 m/s) – but that’s assuming that the falling person is in a typical “spread-eagle” skydiver position, presenting the most surface area to the air that they can. Our Kandorians aren’t. They’re all over the map, from nose-down to tumbling. We need an average value for their terminal velocity, which will be based on the surface area they present to the air as well as their coefficient of drag for their particular shape as they fall.

Upper ranges of terminal velocity run anywhere from 160 mph – 180 mph (nose-down diving, presenting less surface area to the air). Let’s take the middle between 120 mph and 180 mph: 150 mph (67.1 m/s) – as the average speed at which a Kandorian is falling when they reach terminal velocity.

But do they reach terminal velocity during their 1000-meter drop?

This is where the numbers come in. To see if your average Kandorian falling from 1000 m has enough distance to reach terminal velocity, we need to know how long it takes them to reach terminal velocity, and how far they fall in that time. To make things easier, we’re going to ignore air resistance for this part

Air resistance would have the effect of slowing a Kandorian down as they fall to reach terminal velocity, so it would take them longer to reach it, and thus, would fall farther – but let’s see how this works out first. It’s probably a safe assumption.

Let’s start with how long it takes a Kandorian to reach terminal velocity. To do that, we need the definitonal acceleration formula, which is:

Acceleration = change in velocity/change in time, or

a = Δv/Δt

(Δ, the Greek letter “delta,” means “change in,” or, final condition – initial condition)

We know our initial velocity (0 m/s – we’re going to ignore any downward motion that some individuals may have had, and take the initial downward velocity of a Kandorian as zero), our acceleration is -9.8 m/s² (the negative sign means the object is falling down, although we’ll leave it out of our calculations as long as we indicate the Kandorians are falling down), and our final velocity – the terminal velocity of 67.1 m/s.

We rearrange our acceleration formula to solve for time:

t = v_final – v_initial/a

And add in numbers:

t = 67.1 m/s – 0 m/s / 9.8 m/s² = 6.85 seconds.

In other words, it takes a Kandorian, falling from 1000 m, about 7 seconds to reach terminal velocity of 67.1 m/s.

How far do they fall in that time?

We’ve got the time, the velocities (starting and ending), and the acceleration. We can solve for the distance fallen by using the kinematics formula:

Δy = v_it + 1/2at²

The initial velocity again was 0 m/s, so the first term cancels out, leaving us with Δy = 1/2at² or – the distance fallen is equal to one half times the acceleration of the object multiplied by the square of the time the object is falling.

Plugging numbers in:

Δy = (½)(9.8 m/s²)(6.85 s)²

Crunch some numbers, and we get Δy = 229.9 meters.

So – your average Kandorian, flying at 1000 m, who gets hit by Batman’s Green-K rain starts to fall and falls 229.9 meters in 6.85 seconds. At which time, they will be falling at 67.1 m/s – terminal velocity.

That’s it for speed – there’s no falling faster (assuming they maintain their body position, which we will). For the next 770.1 meters, they’ll be falling at a constant velocity of 67.1 m/s. How long will it take from when they reach terminal velocity until they hit the ground? That’s a quick one:

Since speed = distance/time, just rearrange to solve for time, and you get:

time = distance/speed

time = 770.1 m / 67.1 m/s

time = 11.5 seconds

The Kandorians will be falling for about 11.5 seconds at 67.1 m/s, all the while, thinking about all the bad choices they’ve made. Add it to the time it took to accelerate to terminal velocity, and the total fall time for the Kandorians is equal to 18.4 seconds.

What about the hit? How much energy will they hit the ground (or anything else that’s in their way) with?

Since we’re talking about energy, we need to use the work-energy principle. That states, simply, that the work done by an object is equal to the change in energy of an object. Any given Kandorian is the object, and they are falling from a height. Given that they’re in motion, they have kinetic energy (KE), and given that they have height, they also have gravitational potential energy (GPE). Since the Kandorians are moving at terminal velocity, things are a little simpler…and slightly complicated.

On the easy side, since their velocity is a constant 67.1 m/s, their kinetic energy will remain the same for the final 770.1 meters of their fall. On the slightly complicated side is the Kandorian’s gravitational potential energy, or the energy any object has because it has height above the surface is would otherwise be falling towards. The higher the object, the greater the gravitational potential energy, as seen in its formula:

GPE = (mass)(gravity)(height)

Mass is in kilograms, gravity on earth would be 9.8 m/s², and height is measured in meters.

So – as our Kandorians fall, shouldn’t their potential energy decrease?

Yes – and it does.

“But,” you’re saying, “The Law of Conservation of energy says that can’t work. If the Kandorian’s kinetic energy is staying the same at terminal velocity, and the gravitational potential energy is decreasing…that energy has to go somewhere…since it’s not moving into kinetic energy, which is would normally do in a vacuum.”

Wow – good point. But we’re over Gotham City, not in a vacuum. The Kandorians are falling through air, and that air pushes back. The KE of the falling people is constant, and the GPE is decreasing – that energy is dissipated into the air surrounding the Kandorians as they fall, as heat. Falling Kandorians warm the air slightly as they skydive without a parachute for 1000 meters.

So – kinetic energy is what we have to deal with, since gravitational potential energy is not something we need to worry about in this case. How much energy are we talking about for the average Kandorian?

The formula for kinetic energy is a simple one, let’s all say it together:

KE = 1/2mv²

m = mass

v = velocity

Plugging in numbers for our average Kandorian:

KE = ½ (85)(67.1)²

KE = 191,352.4 Joules of energy, per Kandorian.

So what? Joules don’t really make sense as units to too many people outside of physics classrooms, so let’s try to convert a little…

191,352.4 Joules is equal to about 40 grams of TNT. That’s far from the amount you’d need to destroy a vehicle (about 1 kilogram under controlled conditions), but safe to say, if a falling Kandorian hits your car, you’d be talking to your insurance agent, and it’s nothing you’d like to be near. Another equivalent – 191,352.4 J is a little shy of the energy release of a quarter stick of dynamite (250,000 J). Raining Kandorians falling all over your city is like small bombs going off everywhere.

Another way to look at the problems with dropping Kandorians is to look at the force with which they hit cars, roads, building, and the supremely unlucky Gotham City pedestrian. To do that, we need to look at the momentum and impulse of the falling – and stopping – Kandorian.

Momentum describes an object’s resistance to stopping – all moving objects have it, and it can be calculated by multiplying the objects mass by its velocity:

p = (mass)(velocity)

p = momentum. It just is. Yeah, it makes no sense.

Putting numbers in, we find the momentum of an average falling Kandorian to be 5703.5 kg m/s.

To change momentum, you need an impulse, which is a force acting over a period of time, or:

J = FΔt

J = impulse

Δt = the time period during which the force is acting on the object.

Continuing, the impulse-momentum theorem says that the change in momentum of an object is equal to the impulse that is applied to the object. In other words, if you want to change the momentum of an object, you have to apply a force over a period of time.

In our case, if a Kandorian is falling to the ground, the ground is going to change that Kandorian’s momentum (reducing it to zero) very quickly – let’s just say the Kandorian comes to a full stop in 0.1 seconds – a little longer for a surface with “give” (the roof of a car), a little shorter for a surface with less give (concrete).

Before we go to our formula, let’s think of what we have – our Kandorian will have an initial velocity of 67.1 m/s and a final velocity of 0 m/s. The change from really fast to dead stop is 0.1 seconds. The Kandorian’s mass will remain constant.

So, setting this up, we can use the formula that expresses how the impulse is equal to the change in momentum:

FΔt = mΔv

But we only want to know about the force the Kandorian will experience and deliver, so we can solve the formula for force:

F = mΔv/Δt

Plugging in numbers:

F = (85)(67.1)/0.1

(technically, the velocity would be negative (0 – 67.1) which would indicate its direction, down, but we’re going with the notion that we know it’s directed down, and don’t need to put the negative in there this time)

F = 57,035 Newtons, or 12,822 pounds of force.

That’s about the same as a heavy male elephant.

That doesn’t bode well for your car, pavement, buildings, of the guy just running out to grab some milk. Again, simplest form, this is an 85 kg mass smashing into an object at 150 miles an hour. Damage is going to be extensive, and for the residents of Gotham, there would be an extensive period of thunder in the city as body after body smashed into earth.

Appropriately, the comic pages show small explosions throughout the city as the Kandorians impact the buildings and ground around Gotham City. While Batman smiles, of course, noting that the Green-K in rain was dilute, so it’s only going to weaken the Kandorians for a while – long enough for him, Superman, and the angry mob of Gothamites to apply a massive beat-down.

But given the number of Kandorians – a rough count looks to be somewhere between 120 and 150 – and the fact that they were flying in a tightly packed formation…although he saved the city, Batman’s “softening up” of the Kandorians did end up causing Gotham City some fairly widespread property damage.

Which we’re sure the Wayne Foundation is happy to help out with.

One Batman is done beating the tar out of some Kandorians that made the mistake of messing with him.