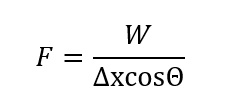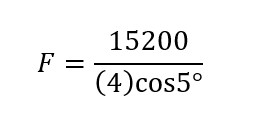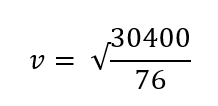The Physics of a Spider-Man Slingshot

Ask any physics teacher, physicist or engineer: we love Spider-Man. With only some minor tweaks, you can make Spider-Man (or one of his major villains) work as an example in pretty much every topic of a general physics course.

Our pal, Assistant Physics Professor and WIRED Dot Physics blogger Rhett Allain has written many articles about Spider-Man’s webs, his motion, swinging vs. running, and that’s just the tip of the webby iceberg.

Seriously – circular motion? Check. Linear vs. angular motion? Check. Electricity? Electro – check. Forces? Check. Biology? Regeneration with The Lizard, implants with Doctor Octopus, DNA modification – he’s got radioactive spider blood. There are literally tons of comics, animation, video games and currently six movies with Spidey action that can be co-opted into a STEM lesson.

What are we talking about today?

We’re here to talk about stretch – specifically what happens when Spider-Man slingshots himself over a distance. It’s a move that Spider-Man has used many, many times in his 40+ year history. Our case study will be the scene from 2004’s Spider-Man 2 when Doctor Octopus has Aunt May held prisoner on the side of a building. Having been knocked across the street, Spider-Man shoots webs at the window frame, pulls himself back, and jumps up. The result? A Spider-Man slingshot. Spidey flies across the street and starts the battle anew with Doc Ock. Aunt May is saved by the way.

Check it out (the good stuff starts around 1:46): 

Shooting himself across the street is a great example of a couple of concepts students run into in first-year physics, namely conservation of energy and projectile motion. There’s a lot of other concepts in there as well, but we’ll just focus on those two for now.

First off – the law of conservation of energy states that total energy in a system remains constant. In other words, energy cannot be created or destroyed. How does that apply here? When Spider-Man pulls back on his webs to “load” his slingshot, he’s giving himself elastic potential energy. He’s stretched an elastic band and he’s the rock. When Spider-Man flies out the window, he has kinetic energy. If we ignore air resistance (and we will – it makes things a little easier for explanations), the principle of conservation of energy says that the magnitude of Spider-Man’s elastic potential energy must equal the magnitude of his kinetic energy. If we can know, we know the other.

So let’s calculate one – the elastic potential energy*.

To keep our energy account all above board, we need to bring in the work-energy theorem. This states that the total change in the kinetic energy of an object must equal the net work done on an object. In other words, Spider-Man’s webs gain elastic potential energy because of the work he does on them. This is work in the physics sense here – a force applied over a distance. At least a part of the force has to be in the same direction of the displacement. Our larger map of this part of the problem – Spider-Man shooting himself out of the window – would then be:

Work_{done by Spider-Man} = Elastic potential energy_{in the webbing} = Kinetic energy_{of flying Spider-Man}

We’re going to need to estimate some numbers here.  

Before we get going – a quick sidenote. If you’re a teacher looking to bring this kind of pop-culture based problem solving into your classroom, or a student looking to tackle something cool, you’re going to have to estimate some values. Not everything is going to be given, or even clear. Be careful – there are rabbit holes within rabbit holes when you start estimating. For this article, I found myself looking up street widths throughout New York City, where the bank the scene was filmed in front of was located (it’s in LA – look for the palm trees and narrow street from street view, and then six lanes when viewed from above). Some numbers will be easier to find than others. Grab what you can, and make reasonable estimates for the others and get on with the problem.

Also – a quick note on my math stylings. You can call it “functional” or “quick and dirty” or “messy” (now you’re just not being nice) – but it gets the job done. You can polish it up when you work up one of these problems.

What can we estimate? Let’s go with a handful of numbers…

Spider-Man’s mass is 76 kg

Spider-Man pulls himself 4 meters backward, away from the window

The street, from building to building, is 33.5 meters wide (a reasonable estimate based on New York street measurements and the fact that the street accommodates six lanes as shown in the film)

Finally, the spring constant of Spider-Man’s webs is 800 N/m, but there are two, so the combined value is 1900 N/m.

Okay – admission time, this last one is a guesstimate. The spring constant of any spring, or spring like material (like webs), is a measure of the spring’s “stiffness.” A stiffer spring is harder to pull back a certain distance, while a loose or less stiff spring is easier to pull back a certain distance. I’m taking a physics teacher benefit here and assigning the value. Look – the properties of Spider-Man’s webs aren’t consistent from movie to movie or even between pages of an issue of a comic book or scenes of a movie. The easiest way around this in problems like this one? I assume that Peter Parker is a genius, and he can his adapt his webs by changes to his webshooters. Need a thinner, stretchier line? A quick flick of a dial before shooting, and you get it. Need a thicker, stiffer webline? Adjust and shoot.

That value it has here actually fits in nicely with what Spider-Man’s webs are supposed to be anyway – synthetic spider-silk. Real spider silk can support about 3,142 Newtons before snapping. All in all, 1900 N/m is an estimate I can live with. It’s a pretty stiff spring (pogo sticks generally have spring constants in the tens of thousands), but I’m okay with that given the thickness of the webbing shown in the film, and the exertion Spider-Man is clearly going through to pull himself back as far as he does. 1900 N/m it is.

Part the First: Stretchy, Stretchy Webs

The big picture of the first part of the problem is the principle of conservation of energy. The total amount of elastic potential energy in Spidey’s webs when he pulls himself back is his total energy budget. The second he lets go of the webs, he’ll have kinetic energy. How much? The exact same amount as he had when his webs were fully stretched out, due to conservation of energy.

How much energy is that? We can use the formula for elastic potential energy:

E_el = ½ kx²

Where:

k = the spring constant (in N/m)

x = Spider-Man’s displacement – how far he pulled himself back, in meters.

Let’s plug in some numbers:

E_el = ½(1900)(4)²

E_el = 15,200 Joules

That’s it – that’s our total energy budget, all Spidey has to work with. Can’t add more or remove any energy without doing work on the system, and we’re not going to. Again, all that energy must transform into Spider-Man’s kinetic energy at the second his hands leave the webs.

How did Spider-Man get that energy? He did the work – he applied a force over a distance when he pulled himself backwards with his webs attached to the windowframe. How much force? We’ve got a formula:

W = FΔxcosΘ

That’s the amount of work done is equal to the force applied multiplied by the distance and the angle between the force and the direction in which the force is applied. From the film, we’re taking that angle to be very small – about 5°. He’s hitting about mid-frame of the window and pulling backwards. There’s a slight downhill slant to his webs when he’s fully pulled back, but not much.  5° works.

So, re-arranging the formula to solve for force:

Put our numbers in:

F = 3819 Newtons

That’s about 850 pounds. It’s like he’s pulling a small-ish horse backward. Which explains the grunting.

Back to our energy conservation and transformation. Again, we’re ignoring air resistance, so as soon as he lets go of the webs, the 15,200 Joules of elastic potential energy is fully transformed into kinetic energy. The formula for kinetic energy is:

E_k = 1/2mv²

Where:

m = Spider-Man’s mass (in kg)

v = velocity (in meters/second)

Re-arranging our formula to solve for velocity, we get:

Plugging numbers in:

v = 20 m/s

Spidey’s flying out of that window and (since we’re ignoring air resistance) across the street at 20 m/s which is about 45 mph. Not bad in a car, but flying, uncontrolled with no means to stop yourself across the street towards a bad guy on a building? That’s not so much fun if you’re not a superhero.

So what have we got?

We’ve got how Spider-Man shoots himself across the street via the conservation of energy. 15,200 Joules is the total energy budget he’s got, so that must transform completely into kinetic energy. From knowing that he shoots out of the window with 15,200 J of kinetic energy, we were able to figure out his velocity. Don’t like any of my estimates? Put your own numbers in there and give it a try.

But this is only half the problem. Once he lets go of those webs, Spider-Man is a projectile. Now we’ve got a projectile problem to deal with.

Part the Second: Spider-Bullet

Let me preface this part by saying that I love special effects in movies. I love the hard work that countless individuals do every day to make the unbelievable look real and amazing.

Okay – so what happens to Spidey after he leaves that window?

The short version, with no math: he didn’t angle his trajectory upward enough.

From the film, Doc Ock and Aunt May are about one story higher than Spider-Man. That’s why we gave him a little bit of an angle earlier – he could see this, and knew that he needed to go up and across, rather than straight across. But that 5° angle? Not nearly enough.

Here’s why – as soon as the webs stopped acting on Spider-Man, he had only one force acting on him: gravity. He’ll be moving horizontally out of the window, but he’ll also be moving down. It’s like the terrific Mythbusters episode which conclusively shows that a bullet dropped and bullet fired hit the ground at the same time. Gravity is the only force acting on the bullet and on Spider-Man, and gravity, lest we ever forget, is an unforgiving mistress.

The good news: Spider-Man would, quickly, make it across the street. Unfortunately, it would be about 30 or so feet below Doc Ock and Aunt May.

And Peter Parker is supposed to be good at math and science.

So let’s do some math and science…

The long version, with math and science and formulas. Oh my.

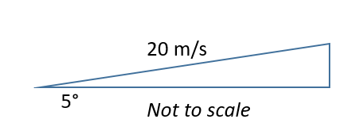
First things first – how long will it take Spider-Man to get to the other building? To figure that out, we need Spidey’s horizontal velocity. This needs a little math. Spider-Man launched from the window at a 5° angle, so he wasn’t perfectly horizontal. His initial velocity across the street had a little bit of vertical and a little horizontal motion in it to make the final motion that was 5° off the horizontal plane. How do we break that down? Trigonometry. Your teacher told you that you’d need it someday.

Think of Spider-Man’s initial velocity of 20 m/s as the hypotenuse of a right triangle (those ones with a 90° angle in them). The angle opposite the right angle is our 5° angle. So, like this:

The vertical and horizontal sides of the triangle represent the vertical and horizontal components of Spidey’s motion. Since we know the magnitude of the hypotenuse and the angle, we can use our trigonometric pals sine and cosine to figure out their respective values. Like this:

Vertical: sin 5° = v_y/20

20(sin 5°) = v_y

v_y = 1.7 m/s

Horizontal: cos 5° = v_x/20

20(cos 5°) = v_x

v_x = 19.9 m/s

So you’re noticing it too, right? Thanks to that small angle, virtually all Spider-Man’s velocity is horizontal.

This is not good.

If we’ve got his horizontal velocity, we can figure out how long it will take Spidey to get acrtoss the street the same way you figure out how long it will get you to go anywhere: divide the distance by the speed. In our case:

time = 33.5 m/19.9 m/s

time = 1.7 seconds

That’s quick.

We’re still ignoring air resistance (if we weren’t Spidey would be slowed down as the air pushed back against his forward motion), but we can’t ignore gravity. Spider-Man’s vertical velocity was upward at 1.7 m/s. Over the course of 1.68 seconds (the time it takes him to get across the street) what happens to him, vertically speaking? Gravity is having its way with him at a rate of 9.8 meters per second, every second, or 9.8 m/s². Where will that put him after 1.68 seconds?

We’ve got a formula.

Δy = v_yit + 1/2at²

That is, the vertical displacement (Δy) is equal to the initial vertical velocity (v_yi) multiplied by time plus the product of one half multiplied by the acceleration (-9.8 m/s²) multiplied by time squared. The acceleration is that due to gravity, and its value is negative because it’s pointed down – up is positive when it comes to vertical motion.

Plug those numbers in:

Δy = (1.7)(1.7) + 1/2(-9.8)(1.7)²

Δy = 2.9 – 14.2

Δy = -11.3 meters.

Um.

That means that Spider-Man would reach the building (in 1.7 seconds) 11.3 meters below where he started.

So what happened, and how could Spidey have made this all work?

Part the Third: Doomed by the Physics

Spider-Man’s trajectory is all wrong for what’s shown. Kudos to the special effects folks for making him streamline himself out after he left the window to reduce air resistance, but still…he’s shown traveling upward toward Dock Ock. There’s no way that can happen unless he’s got a motor strapped to his feet.

Now I know what you’re thinking – could it work? Did the filmmakers just get some of the angles and measurements wrong?

Basically – no. Change the numbers, change the angle, it just won’t work within the confines of the set as it was shown.

I’m going to play teacher here (it’s what I do), so I’ll just point you in the direction you need to go and you can do the work to prove it.

To reach Dock Ock and Aunt May, Spider-Man needed a value of 3 meters for Δy. That is, three meters above his horizontal position. Put 3 in for Δy above and solve for v_yi. You should get around 10.1 m/s.

Take that and go back into the triangle from which we got our horizontal and vertical velocities. Use tangent and solve for your launch angle. Should be about 30°.

Okay – sounds do-able, they just messed up the angle at which he attached his webs to the window frame.

Do that one yourself. If Spider-Man pulled himself back 4 meters, and the angle of launch needed to be 30°, use tangent to find far down Spidey needed to launch from. Should be about 2 meters and change. Spider-Man was in a room. There wasn’t any space below him – well, maybe he could have pulled those webs about half a meter lower, but that’s not going to give him the 30° he needs. Maybe – just maybe, he could attach his webs at the very top of the window frame and manage to get that 2+ meters and thus the 30°, but he’d have to be careful going through that window or he’d do Doc Ock’s job for him with a quick decapitation.

Now I know what you’re still thinking…but no. Increase the spring constant of the webs, right? You still need the angle. And besides, the only effect changing the k value would have is to increase the total elastic potential energy as he pulls back those 4 meters. That, now larger, value transforms into kinetic energy. Spidey’s mass isn’t changing, so his velocity would have to make up for the difference. A larger velocity means he’s getting across the street faster, which is…okay, but he was already traveling at about 45 mph. increase that speed too much, and you start brushing up against the average human response time of 0.25 seconds, which means Dock Ock wouldn’t have time to do…anything as his brain processed this Spider-Man shaped bullet shooting across the street at him.

And we never even talked about how Spider-Man would stop himself.

But in the end, while the physics and math don’t support the entirety of this example of Spider-Man sling shotting himself, it’s still a great scene.

How would you recommend to Spider-Man (or his storytellers) how he could better use the slingshot method of getting from here to there? Can you make an example that works? Keep a careful eye on your spring constant, your angle and your final vertical position – they’ll get you every time. 

 (*) – Yes, we could have started from the other side and calculated the kinetic energy, figuring Spider-Man’s velocity by timing how long it took him to fly across the street, but the frame rate wasn’t consistent to my eye. He appeared to leave the window slowly, there was a cutaway to Doc Ock popping the spike in his arm, and then the slow motion when Aunt May clocked him with her umbrella. All in all, it takes Spidey about ten seconds to get across the street. Find a street, and count out ten seconds while your eyes tracks across it from right to left. That’s super slow.  You’d be falling more than moving horizontally.

Special thanks to Dan Simpson for suggesting this topic an embarrassingly long time ago.